Cosmic shear results from the deep lens survey - I: Joint constraints on omega_m and sigma_8 with a two-dimensional analysis PDF
Preview Cosmic shear results from the deep lens survey - I: Joint constraints on omega_m and sigma_8 with a two-dimensional analysis
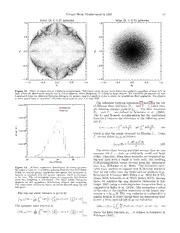
Draftversion October 11,2012 PreprinttypesetusingLATEXstyleemulateapjv.5/2/11 COSMIC SHEAR RESULTS FROM THE DEEP LENS SURVEY - I: JOINT CONSTRAINTS ON Ω AND σ M 8 WITH A TWO-DIMENSIONAL ANALYSIS M. JAMES JEE1, J. ANTHONY TYSON1, MICHAEL D. SCHNEIDER1,2, DAVID WITTMAN1, SAMUEL SCHMIDT1, STEFAN HILBERT3 Draft versionOctober 11, 2012 ABSTRACT 2 We present a cosmic shear study from the Deep Lens Survey (DLS), a deep BVRz multi-band 1 imagingsurveyoffive 4 sq. degreefields with two NationalOpticalAstronomyObservatory(NOAO) 0 4-meter telescopes at Kitt Peak and Cerro Tololo. For both telescopes, the change of the point- 2 spread-function (PSF) shape across the focal plane is complicated, and the exposure-to-exposure t variationofthisposition-dependentPSFchangeissignificant. Weovercomethischallengebymodeling c the PSF separately for individual exposures and CCDs with principal component analysis (PCA). O We find that stacking these PSFs reproduces the final PSF pattern on the mosaic image with high fidelity, and the method successfully separates PSF-induced systematics from gravitational lensing 9 effects. Wecalibrateourshearsandestimatetheerrors,utilizinganimagesimulator,whichgenerates sheared ground-based galaxy images from deep Hubble Space Telescope archival data with a realistic ] O atmospheric turbulence model. For cosmological parameter constraints, we marginalize over shear calibration error, photometric redshift uncertainty, and the Hubble constant. We use cosmology- C dependent covariances for the Markov Chain Monte Carlo analysis and find that the role of this . h varying covariance is critical in our parameter estimation. Our current non-tomographic analysis p aloneconstrains the Ω −σ likelihoodcontourtightly, providinga joint constraintof Ω =0.262± M 8 M - 0.051 and σ = 0.868±0.071. We expect that a future DLS weak-lensing tomographic study will o 8 furthertightentheseconstraintsbecauseexplicittreatmentoftheredshiftdependenceofcosmicshear r t more efficiently breaks the ΩM −σ8 degeneracy. Combining the current results with the Wilkinson s Microwave Anisotropy Probe 7-year (WMAP7) likelihood data, we obtain Ω = 0.278±0.018 and a M σ =0.815±0.020. [ 8 Subjectheadings: cosmologicalparameters—gravitationallensing: weak—darkmatter—cosmology: 1 observations — large-scale structure of Universe v 2 3 1. INTRODUCTION The Dark Energy Survey Collaboration2005), the KIlo- 7 Degree Survey (KIDS; Verdoes Kleijn et al. 2011), the Weak gravitational lensing from large-scale structures 2 PanoramicSurveyTelesopeandRapidResponseSystem in the universe, often called cosmic shear, allows one 0. to address a number of critical issues in modern cos- (Pan-STARRS,Kaiseretal. 2010),etc. Next-generation 1 mology. Its application encompasses the study of the weak-lensing projects are the Euclid mission (Laureijs 2 universe’s matter density and its fluctuation, probes of et al. 2010), the Wide Field Infrared Survey Telescope 1 (WFIRST; Green et al. 2011), and the Large Synoptic the footprints of non-Gaussianity in the primordial den- : Survey Telescope (LSST, LSST Science Collaborations v sity fluctuation, constraints on dark energy and its evo- et al. 2009). i lution, tests for modified gravity, etc. The consensus X Needless to say, greateffort shouldbe givento control on the critical role of cosmic shear studies triggered of systematics in both shear and photometric redshift r quite a few optical surveys such as the Canada-France- a measurements for these future surveys. The unprece- Hawaii-Telescope Legacy Survey (CFHT-LS; Hoekstra dentedly small statistical errors will bring revolutionary et al. 2006, Semboloni et al. 2006; Fu et al. 2008), advances to cosmology only if progress in shear cali- the Red-sequence Cluster Survey (RCS; Gladders et bration and control of catastrophic errors in photomet- al. 2003), the Cerro Tololo Inter-American Observa- ric redshift estimation parallels the increase in statisti- tory (CTIO) Lensing Survey (Jarvis et al. 2006), the cal power. The recent shear estimation challenges such Garching-BonnDeep Survey(GaBoDS, Hetterscheidt et as the Shear TEsting Programme (STEP, Massey et al. al. 2007), the VIRMOS-DESCART survey (VIRMOS, 2007; Heymans et al. 2006), the GRavitational lEnsing VanWaerbekeetal. 2005),the DeepLensSurvey(DLS, Accuracy Testing (GREAT, Bridle et al. 2009; Kitching Tyson et al. 2001, Wittman et al. 2006), etc. The etal. 2012),etc. areconcertedeffortstoquantifybiasin current surveys include the Dark Energy Survey (DES, the current popular shear estimation methods and also 1DepartmentofPhysics,UniversityofCalifornia,Davis,One toidentifythelimitationofthecurrentweak-lensingsim- ShieldsAvenue,Davis,CA95616 ulation methods. Similar efforts toward improvement of 2Lawrence Livermore National Laboratory, P.O. Box 808 L- photometric redshift estimation, albeit less mature, are 210,Livermore,CA94551 also underway (e.g., Hildebrandt et al. 2010). 3Kavli Institute of Particle Astrophysics and Cosmology Both sky coverage and depth must be carefully bal- (KIPAC), Stanford University, 452 Lomita Mall, Stanford, CA 94305, and SLAC National Accelerator Laboratory, 2575 Sand anced to maximize the scientific return from future cos- HillRoad,M/S29,MenloPark,CA94025 mic shear surveys. Large sky coverageis needed to min- 2 Jee et al. cosmic shear signal. The structure of this paper is as follows. In §2, we de- scribe our DLS data and analysis method including our detailedPSFmodelingandshearcalibrationefforts. The theoretical background of cosmic shear and our system- atics control is presented in §3. We discuss the study of cosmologicalparameterconstraintsin§4andconcludein §5. 2. OBSERVATIONS 2.1. Data The detailed description of the DLS4 can be found in Wittman et al. (2006; 2012). Below we provide a brief summary of the survey and its data. The DLS covers five 2◦ ×2◦ fields (hereafter F1-F5). F1andF2areinthenorthernsky,andobservedwiththe KittPeakMayall4-mtelescope/MosaicPrime-FocusIm- ager (Muller et al. 1998). F3, F4, and F5, which are in the southern sky, were observed with the Cerro Tololo Blanco 4-m telescope/Mosaic Prime-Focus Imager. Ta- ble 1 lists the coordinates of the five fields. Figure 1. Surveyareaanddepthofvariousopticalsurveys. The red line represents the AΩt = constant locus, where A, Ω, and EachMosaicImagerprovidesa∼35′×35′fieldofview t are the primary mirror area, field of view, and exposure time, with a 4×2 array of 2 k× 4 k CCDs (∼0′.′26 per pixel). respectively. DLSisthedeepestoptical surveytodateamongthe We divide each 2◦ × 2◦ DLS field into a grid of 3×3 current &10sq. degree surveys. Depth iscompared either inthe array. Each 40′ ×40′ subfield, slightly larger than the Roriband. camera field of view, was covered with dithers of ∼200′′. The DLS data consists of 120 nights of B, V, R, and imize the contributionto the errorfromthe samplevari- z imaging. A priority was given to the R filter, where ance. Deep imaging is required to detect and measure we measure our lensing signal, whenever the seeing was the shapes of high redshift sources, which allows us to better than ∼0′.′9. The mean cumulative exposure time probethe evolutionofthe cosmicstructureovera signif- inRisabout18,000sperfieldwhereasitisabout12,000s icant fraction of the age of the universe. The Deep Lens per field for each of the rest of the filters. The typical Survey (DLS; Tyson et al. 2001, Wittman et al. 2006) exposure time per visit is about 900s. is designed as a precursor to these next generation cos- mic shear surveys with emphasis on the latter, reaching 2.2. Reduction a mean source redshift of z ∼ 1 over 20 sq. degrees us- ing the two National Optical Astronomical Observatory We applied initial bias, flat, and geometric distor- (NOAO) Mayall and Blanco 4-meter telescopes. Fig- tion correction to the DLS data with the IRAF package ure 1 shows the comparison of DLS sky coverage and MSCRED.External astrometric calibration was performed depth with those of other optical surveys. DLS is the bymatchingastronomicalobjectsineachexposuretothe deepest optical survey to date among the current & 10 USNO-B1 star catalog using the msccmatch task. The sq. degree surveys. Galaxy populations are dominated residual uncertainty in the global coordinate system rel- by faint blue galaxies when a survey reaches or exceeds ative to the USNO-B1 catalog is less than 0.01′′. The the depth of the DLS. As no ground-basedcosmic shear mean rms error per object is ∼0′.′3. The limiting factor study with a comparable depth has been presented, the for this scatter per object is believed to be the internal currentcosmic shear analysis with the DLS is an impor- accuracy of the USNO-B1 catalog. Internal astrometric tant experiment, testing whether the shapes of the faint calibrationbetween different epoch data was carriedout blue galaxy population smeared by atmospheric seeing usingthecommonhighS/Nstarspresentintheoverlap- can be reliably used for cosmic shear. We augment this ping region. Precise registration is essential in precision experimentusingimagesimulationswithrealgalaxyim- weak-lensing analysis because a small ∼0.5 pixel error ages. In addition, our seeing-matched photometry from can create a noticeable correlation of object ellipticity thedeepBVRzimaginginconjunctionwithalargespec- overalargescale. Weverifythatthemeanrmserrorper troscopic sample allows us to stabilize our photometric objectis less than∼0.1pixelandthe scatteris isotropic, redshift estimation and to identify where potential sys- which indicates that the scatter is dominated by photon tematic errors lie in our results. Reliable photometric noise. redshifts arepivotalnotonly inthe interpretationofthe We found an initial non-negligible (10−20%) residual cosmic shear signal, but also in future application of the flatfielding error in the final stack image after the appli- measurements to weak lensing tomography. cation of the sky-flat correction. This is further refined This paper is the first in a series of our DLS cosmic tothe2−5%levelusingthe“u¨bercal”method(Padman- shear publications. Here we mainly focus on the DLS abhan et al. 2008). Interested readers are referred to systematicsinducedbythepoint-spread-function(PSF), Wittmanetal. (2012)fordetailsofour“ubercal”imple- the removal of the systematics with our principal com- mentation and performance. ponentanalysis(PCA)and“StackFit”methods,andthe two-dimensional (non-tomographic) analysis of the DLS 4 http://dls.physics.ucdavis.edu. Cosmic Shear Measurement in DLS 3 Table 1 DLSFieldsandData FieldName RA DEC MedianSeeing(R) z¯source nsource (”) (persq. arcmin) F1 00:53:25 +12:33:55 0.96 0.93 13.3 F2 09:18:00 +30:00:00 0.85 1.07 20.5 F3 05:20:00 –49:00:00 0.87 1.15 16.0 F4 10:52:00 –05:00:00 0.87 1.08 14.3 F5 13:55:00 –10:00:00 0.86 1.07 15.8 The spatial variation of the PSF is substantial and complicated for both the Mayall and Blanco telescopes. Anexampleofthis PSFpatternisdisplayedinFigure2. Althoughthisparticularpatternisobservedon24Febru- ary 2001 from the Blanco telescope, a similar degree of PSF variation complexity is commonly present in all of our DLS data. It is difficult to interpolate the variation overthe entire focal plane with a single set of polynomi- als. Thus, polynomialinterpolation shouldbe limited to asmallerarea,wherethevariationisslowandtractable. Hence,wechoosetomodelthePSFvariationonaCCD- by-CCD basis. This chipwise approach was investigated by Jee et al. (2011) for the LSST, where the small f- ratio of the optics makes the potential aberrationhighly sensitive to CCD flatness, giving rise to a sudden, no- ticeable jump in PSF patterns across CCD boundaries. For the Mayall and Blanco telescopes, we often found a somewhat smaller, but clear discontinuity across the CCD gaps, although in principle the relatively large f- ratioofthetwotelescopesshouldmaketheCCD-to-CCD flatness much less important. Asmostlensingsignalscomefromdistant,faintgalax- Figure 2. Example of spatial variation of DLS PSF. Although ies, which sometimes are not even detected in single ex- this particular pattern is observed on 24 February 2001 from the posures, these source galaxies are commonly examined Blanco telescope, a similar degree of PSF variation complexity is aftermulti-epochdataarecombinedtoproducethedeep commonlypresentinallofourDLSdata. Each“whisker”showsthe stack image (i.e., single 900s exposure vs. cumulative directionandmagnitudeofthestellarellipticityatthelocationby itsorientationandlength,respectively. Theredstickinthemiddle 18,000s exposure). Therefore, it is important that the shows the size of 10% ellipticity [i.e., (a−b)/(a+b)= 0.1]. The PSF modeling closely mimics the image stacking proce- eightshadedrectanglesdepicttheeightCCDsofthecamera. Here dure (e.g.,offsets, rotations,geometricdistortioncorrec- wedidnotcleanupoutliers(e.g.,cosmic-rayhitstars,binarystars, tions,etc.). Figure3schematicallyillustrateshowimage etc.),andtheydonotrepresentrealPSFs. stacking complicates the PSF pattern. After stacking is Our team has developed two pipelines (Pipeline I and performed, across the image boundaries of input frames II) for the creation of the final mosaic. Pipeline I is we often observe a discontinuous change of PSF as dis- optimized for photometry and consists of independently played. This discontinuity prohibits us from interpolat- implemented standalone programs (Wittman et al. in ing PSFs based on the information obtained only from preparation). It performs PSF-matched photometry to the final stackedimage. Hence, inour DLS weaklensing minimizethesystematicsinphotometricredshiftestima- analysis,thePSFmodelingisperformedwiththefollow- tion (Schmidt et al. in preparation). Pipeline II is opti- ing two steps to address the issue. First, we construct a mizedforweaklensingandcontrolstheflowoftheSCAMP PSF model for each CCD image using a PCA method. andSWARPprograms5. WeprocessonlyR-banddatawith Then, the PSF on the final mosaic image is computed thissecondpipeline. Thesetwopipelinessharetheabove by weighted combination of all contributing PSFs from procedures, but differ in that the weak-lensing pipeline each CCD image. Below we provide the details for each uses the subset (with better seeing and less astrometric step. issues)oftheDLSdataandcreatesalarge2◦×2◦mosaic image per field whereas Pipeline I produces nine (3×3) 2.3.1. Step 1: PSF Modeling with PCA for each CCD image subfieldimagestocovereach2◦×2◦ field. In§2.3wede- A 2◦ ×2◦ mosaic image for each DLS field is created scribe this weak-lensing pipeline in detail in the context withtheSCAMP/SWARPsoftware. TheSCAMPprogramau- of the PSF reconstruction. tomaticallyrefinesWCSheadersofimagesbyfirstcross- identifying astronomical objects with external standard 2.3. PSF Reconstruction catalogs and then by tweaking the WCS information of each header in such a way that internal consistency is 5 availableathttp://www.astromatic.net. maximized. Because the astrometric solution is already 4 Jee et al. Figure 3. ComplicationinPSFmodelingduetoimagestacking. Weak-lensinganalysisistypicallyperformedonastackedimage,which oftenexhibits sharpPSFdiscontinuities. Thefigure schematicallyshows how thiscomplication arises. When wecombine the twoimages intheleftpanel,theresultingPSF(right)possessesabruptellipticitychanges acrosstheboundariesofinputframes. obtainedinthe photometricpipeline tothe weak-lensing precision, we feed the Pipeline I catalogs into SCAMP as an external catalog. The Swarp program utilizes the series of these refined WCS headersto define the globalWCS for the final mo- saic. Then, the input images are resampled and com- bined to create the final mosaic. We use the Lanczos3 interpolation kernel, which mimics the ideal sinc kernel andis knownto suppress the correlationbetweenpixels. We estimatethatthe covariancebetweenadjacentpixels is about 7% of the variance. This inter-pixel correlation leads to underestimationof both photometric errorsand shapeerrors. Theslightshiftinshapeerrorsalsochanges theweightinourshearcorrelationcomputation. Inprin- ciple, wecanremedythe situationby increasingour rms map to compensate for this underestimation. However, we conclude that this step is unnecessary because the resulting change in weight distribution is small and well within the interval of the shear calibration marginaliza- tion (§2.5.2). What we should potentially be concerned about is the Figure 4. Variance vs. number of PSF basis functions systematics (multiplicative) in shear calibration. The (eigenPSFs). To determine the number of basis functions for a inter-pixel correlation somewhat smears the galaxy pro- compact description of the PSF, weexamine fractional data vari- ancefordifferentnumberofbasisfunctions. ForHST/ACSPSFs, file and on average circularizes the shapes. Fortunately, we observe that the growth slows down notably after ∼20 (Jee et since we use the same Lanczos3 kernel in image simu- al. 2007). For the PSF of the 4-m Mayall/Blanco telescopes, this lations for our shear calibration (§2.5.2), the resulting happensat∼5. Thesimplerprofileoftheground-basedPSF(asop- multiplicative factor already includes this effect. posedtocomplex,diffractionlimitedPSFofHST)requiresfewer basis functions. However, because of the larger FWHM variation The Swarpprogramprovidesanoptionto keepthe in- (i.e.,atmosphericseeing),thetotalvarianceremainsslightlylower termediateresampledimages(hereafterRESAMPimages). thaninthecaseofHST/ACS(∼96%vs. ∼99%at20). ThePSF We use these RESAMPimages to identify stars and model reconstructionoftheMayall/BlancoTelescopesdoesnotshowany PSFs because they are properly rotated, shifted, and significantdifferenceinqualityaslongasthenumberofeigenPSFs is≫5. Inthecurrentstudy,wechoosetokeep20eigenPSFs. distortion-corrected. For some unknown reason, some frames are found to possess rather large (&0.2 pix) sys- tematic offsets with respect to the stacked image. In two-parameter space iteratively for the stellar locus in addition,the PSFofsome frames aresignificantlylarger the half-light radius range from 1.4 pixels to 5.5 pixels than our criterion (FWHM=1′′). About 5% of the data and the magnitude range whose minimum value (maxi- fall into this group, and we exclude these images for the mum flux) is adjusted depending on the saturationlevel creation of the final stack. of the input frame. We discard stars whose SExtractor The2◦×2◦ mosaicimageforeachfieldconsistsofmore (Bertin&Arnouts1996)flagsarenotzero. Theresulting than ∼ 1,200 CCD images. Consequently, we need an “clean”starsareusedtoderivetheprincipalcomponents automaticproceduretoselecthighS/Nisolatedstarsand (eigenPSF), and the coefficients (i.e., amplitude along apply PCA to them. Our star-selection algorithm relies the eigenPSF) are computed. To determine the number on the size versus magnitude relation with some impor- of basis functions for a compact description of the PSF, tant fail-safe procedures. The algorithm starts with an weexaminefractionaldatavariancefordifferentnumber initialguessofthehalf-lightradiusandmagnituderange ofbasisfunctions(Figure4). Thetotalvariancedoesnot of the “good” stars. Of course, because of the varia- increase rapidly after five, and thus the choice is some- tion in telescope seeing and exposure time, the stellar what arbitrary. We choose to keep 20 eigenPSFs, which locus shifts exposure by exposure. Thus, we search the accounts for ∼96% of the total variance. Afterweobtainthese20eigenPSFs,thekth starimage Cosmic Shear Measurement in DLS 5 is decomposed as lower ellipticity by δe = 0.001 ∼ 0.003 with respect to the data PSF. This is because the procedure in the nmax PSF sampling from noisy stars slightly circularizes the C (i,j)= a P (i,j)+T(i,j), (1) k kn n model PSF. Using this imperfect PSF model for our nX=0 galaxyshapemeasurementleadstonon-negligibleunder- where C (i,j) is the normalized pixel value of the kth correction. Hence,wecompensateforthiscircularization k byincreasingtheellipticity(withoutalteringtheposition star image at the pixel coordinate (i,j), P is the nth n angle) of the model PSF by δe = 0.001 ∼ 0.003. This eigenPSF, a is the projection of the kth star in P , kn n “re-stretching” is implemented by shearing the PSF im- and T is the mean PSF. Because P ’s are orthogonal n age in real space, and the applied shear is a constant to one another, one can determine a by multiplying kn (fixed for each DLS field) fraction of the PSF ellipticity. thecorrespondingeigenPSFtothemean-subtractedstar The exact amount of re-stretching for this first-level image. tweakingisdeterminedusingthefollowingtwodiagnostic Approximately50-200starsareavailableper CCDper functions proposed by Rowe (2010): exposure depending on galactic latitude, and we fit 3rd order polynomials to the spatial variation of the coeffi- D (r)≡h(e −e )∗(e −e )i(r) (2) 1 d m d m cients to enable interpolation at any arbitrary position D (r)≡he∗(e −e )+(e −e )∗e i(r) (3) within the CCD. When we experiment with 4th order 2 d d m d m d polynomialsinstead,theinterpolationbecomesoccasion- wheree ande aretheellipticityofthedataandmodel ally unstable for some frames, where the number ofhigh d m PSFs,respectivelyincomplexnotation(see§2.5.1). Con- S/N stars is not sufficient. In addition, we find that the sequently, D and D show the residual autocorrela- interpolation by 2nd order polynomials slightly underfit 1 2 tionandthedata-residualcross-correlation,respectively. the spatial variation with respect to the 3rd order poly- Rowe (2010) suggests that a combined use of these two nomial result, increasing the amplitude of the residual functions provides an insight into systematics of the correlationby10%-20%. ThePSFsolutiononeachCCD model. In Figure 7 we display D (r) and D (r) for F2. on each exposure is iteratively refined by comparing the 1 2 The left panel displays the result directly obtained from model PSF with the observed star and eliminating sig- ourPSFstackingwhereasthemiddlepanelshowsthere- nificant outliers. sultwhenthisPSFmodelontheleftpanelisre-stretched tocompensateforthePSFcircularization. Theimprove- 2.3.2. Step 2: PSF STACKING ment is more noticeable in D (r). When comparing the 2 The PSF models for individual RESAMP images are amplitudes of D and D , one should remember that 1 2 inverse-variance weight-averaged to create the PSF on D (r) is in generalmore sensitive to the presence of sys- 2 the mosaic image, where the weak-lensing signal is mea- tematics than D (r) in part because D (r) is a sum of 1 2 sured. The image header of the RESAMP file contains the two data-residual ellipticity correlation functions (in or- shift information (i.e., integer offsets). For each object, der to cancel the imaginary part), and in part because we need to loop over the list of the RESAMP files to stack theellipticityofthePSFishigherthanthatoftheresid- PSFs. In order to determine whether or not anobject is ual. Forotherpossiblereasons,wereferreaderstoRowe observed by a given RESAMP image and also to find the (2010). exactweightvalueusedinco-adding,weutilizethecorre- The small residual correlation functions in the middle sponding“projected”weightmapgeneratedbySwarp. If panel of Figure 7 suggests that the above re-stretched theobjectisfoundtobewithintheweightmap,wecom- PSF model is an excellent description of the data. How- pute the PSFatthe shifted location(rememberthatthe ever,wenoticethatthe shapesofgalaxiesobtainedwith RESAMPimagesarealreadyrotatedtoproperlyalignwith this re-stretched PSF (middle) tends to be still under- the final stack) and applied the corresponding weight. corrected. In other words, collectively speaking, galaxy Figure 5 and 6 illustrate that the above PSF stacking shapes are still biased toward the initial anisotropy of schemecloselyreproducestheobservedellipticitypattern the PSF. We suspect that this phenomenon is in part in F2. In Figure 5, the whiskers show the ellipticity dis- relatedtotheso-calledcentroidbiasmentionedbyBern- tribution ofthe stars directly measuredfromthe 2◦×2◦ stein & Jarvis (2002) and Kaiser (2010). According to mosaic image. The PSF ellipticity change pattern mim- theseauthors,evenaperfectPSFmodelwillnotremove icsthe 3×3pointingpatternofthe DLSobservation. In the PSF bias completely because the centroid of the ob- addition,itiseasytoseethatacrosstheexposurebound- ject is more uncertain along the elongation of the PSF. aries (where the level of the shade changes) the PSF el- This bias does not go away even if we treat the centroid lipticityoftenexhibitsasuddenchange. Thewhiskersin as free parameters. This is the reason that we need a Figure 6 display the ellipticity of the model PSFs evalu- second-leveltweakingmentionedabove. We addressthis ated (interpolation + stacking) at the location of these issue by further stretching the model PSF so that the stars. Thesimilarityinboththesizeanddirectionofthe ellipticity increases by additional hδei ∼ 3×10−4. The whiskers across the entire field is remarkable. amount of this additional stretching is also a fixed (for Despite this seemingly nice agreement in the PSF on each DLS field) fraction of the PSF ellipticity, and the thestackedimage,however,wefindthatthisinitialPSF first-order value is determined mainly utilizing our im- model must be “tweaked” to remove the PSF-induced age simulations, where galaxies are randomly oriented anisotropy to our satisfaction. This tweaking is carried (i.e., no shear is present). We adjust the stretching fac- out in two steps, for which we provide the details as toruntil the PSF-induced residualshear signalvanishes. follows. Then, we refine this factor by making sure that the am- First, the model PSF tends to have systematically plitude of star-galaxy correlations (§3.2.1) and B-mode 6 Jee et al. Figure 5. Observed PSF ellipticity in the stacked image for F2. The whiskers display the ellipticity distribution of the stars directly measuredfromthe 2◦×2◦ mosaicimage. Thebackground shade represents the weightmap (darker shade indicates lower value) derived from both exposure maps and photon statistics, and illustrates the complexity of the weight distribution. PSF discontinuities occur at exposureboundaries(i.e.,atdiscontinuitiesintheweightmap). Wedidnotcleanupoutliers(e.g.,cosmic-rayhitstars,binarystars,etc.), andtheydonotrepresentrealPSFs. signals (§3.2.2) also decreases simultaneously. The right It is obvious that the PSF model that we obtain from panel of Figure 7 shows the resulting D (r) and D (r) the second tweak gives the smallest amplitude for star- 1 2 diagnostic functions when this second-tweak is applied galaxy correlations, although the amplitude of the di- tothe first-tweakPSFmodelshowninthemiddle panel. agnostic function (especially D ) of this PSF is not the 2 NotethatthisincreasesthedeviationofD (r)fromzero smallest. 2 at 8′ . θ . 70′, although this final PSF removes the One should not be misled into thinking that our PSF- PSF-induced anisotropy from galaxy images most satis- tweaking removesany arbitraryB-mode signal. System- factorily among the three cases shown here. Figure 8 atics arising from non-centroidbias cannot be made dis- displays the star-galaxy correlation functions (see §3.2.1 appearbysimplyincreasingtheellipticityofeverymodel forthedefinitions)forthe threecasesshowninFigure7. PSF uniformly by a constant factor. In addition, the Cosmic Shear Measurement in DLS 7 Figure 6. PCA PSFreconstruction inthe stacked image forF2. Thewhiskers show the ellipticityof the PSFs evaluated (interpolation + stacking) at the location of the stars inFigure5. The agreement between observation and model inboth the sizeand direction of the whiskersacrosstheentirefieldisremarkable. above PSF-tweaking cannot arbitrarily get rid of intrin- Kaiser, Squires, & Broadhurst (1995) and Fischer & sic alignment signals. Tyson (1997), and many variations exist. The later al- gorithmsapproximatethesurfaceprofileofgalaxieswith 2.4. Galaxy Ellipticity Measurement some analytic profiles. These analytic profiles are con- volved with PSF models before being fit to the images There exist a number of algorithms for galaxy shape rather than fit to a deconvolved image. While the clas- measurement in the context of weak lensing. Depend- sic,moments-basedmethods continue to be popular and ing on how one approaches the issue for the removal updated, cosmic shear studies are relying more on the of PSF effect, we can classify the existing algorithms second, profile-fitting approach to overcome the poten- into moments-based methods and profile-fitting meth- tial limitations (Kaiser 2000) of the moments-based ap- ods. The former methods measure second-moments for proach. both galaxies and PSFs and use them to estimate the Our shape measurement algorithm belongs to the sec- pre-seeing ellipticity. This approach was pioneered by 8 Jee et al. Figure 7. Diagnosticellipticitycorrelationfunctions forPSFmodeling. D1(r)andD2(r)aretheresidualautocorrelation andthe data- residualcross-correlation,respectively(Rowe2010). Anysignificantdeparturefromzeroindicates thatthemodelpossessesnon-negligible systematics. Here we show the case for F2. The left panel displays the result directly obtained from our raw PSF model whereas the middle and right panels shows the results obtained after the application of the first-level and second-level corrections, respectively. The firsttweakisneededtoimprovethePSFellipticityagreementbetweenthemodelanddata. Thisisdonebyincreasingtheellipticityofthe model PSF by 0.001−0.002. However, this first-tweak model does not remove the PSF-induced anisotropy in galaxy images completely due to the centroid bias. We have to further increase the ellipticity of the first-tweaked PSF by ∼3×10−4 to remove this residual bias; notethatthismakesD2(r)deviatesfromzeroat8′.θ.70′. OneshouldrememberthatD2(r)isingeneralmoresensitivetosystematics thanD1(r)inpartbecausetheintrinsicellipticityofthePSFismuchlargerthantheresidualPSFellipticity(seeRowe2010forextensive discussionontheissue). Figure 8. Star-galaxycorrelation asdiagnostics of PSFmodel. We show the caseforF2. Theleft, middle,and rightpanels correspond to the PSFmodels showninthe left, middle, and rightpanels inFigure7. Filledand open circlescorrespond to the “tt” and “××”(see §3.1forthedefinition)correlations,respectively. ond category. We fit a PSF-convolved elliptical Gaus- for the centroid. We fix the centroid6 and the back- sianto a galaxyimage. Ofcourse,anelliptical Gaussian groundusing the SExtractor’sxwin image, ywin image, profile is not the best approximation of galaxy profiles. andbackgroundsothatthe totalnumberoffreeparam- This sub-optimal fitting is termed “underfitting” (Bern- eters is only four, which further stabilizes the minimiza- stein 2011) and has been shown to cause some bias in tion and reduces the ellipticity uncertainty. The initial shearestimation. However,we find that this bias is only guessesfor these four parametersare computed utilizing multiplicative and thus can be calibrated out with care- SExtractor measurements. ful image simulations (discussed in §2.5.2). Our experi- For each object, square postage stamp images are ex- ments with S´ersic profiles show that although this mul- tractedfromthefinalstackandrmsmap. Wechoosethe tiplicativefactorisreduced,themeasurementuncertain- sizeofthispostagestampimagetobe(8a+20)pixelson ties increase. This increase in ellipticity uncertainty is aside,whereaisthesemi-majoraxisinitiallydetermined attributedtothefollowingtwofacts. First,S´ersicprofile by SExtractor. In most cases, the image contains pixels fitting takes into account more pixels farther from the belonging to other objects and we need to mask them object center, introducing larger noise. Second, S´ersic out. This is implemented by replacing the rms values of profilefitting involvesmorefreeparameterstomarginal- thesepixelswithverylargenumbers,thusmaskingthem ize over. We want to include as many faint galaxies as outinfurtherprocessing. Theidentificationofthesepix- possible for shear measurement as long as the net noise els is based on the information in the segmentationmap (quadratic sum of systematic and statistical noise) goes output by SExtractor. The shape measurement code is down, and we find that using Gaussian over other more written in IDL, and the MPFIT7 module was employed sophisticatedprofilesincreasestheoverallS/Nofourcos- as a minimizer. MPFIT estimates parameter uncertain- mic shear signal. ties from a Hessian matrix. We convert these errors to Formally,adescriptionofagalaxyimagewithanellip- ellipticity uncertainties by error propagation. tical Gaussian requires the following seven free param- eters: normalization, semi-major and semi-minor axes, 6Whenwefreetheobjectcentroid,thenumberofusablegalaxies position angle, background level, and two parameters decrease by ∼8% and the multiplicative shear calibration factor increasesby3−6%. 7 availableathttp://www.physics.wisc.edu/∼ craigm/idl/. Cosmic Shear Measurement in DLS 9 2.5. Shear Estimation our weak-lensing image simulations for bright (R < 22) 2.5.1. Shear Estimator galaxies, but gradually underestimates the shear dilu- tioneffectas the S/Nofthe objects decreases. OurDLS Gravitational lensing transforms the shape in the shear calibration hereafter is purely based on our image source plane to the image plane according to the fol- simulation studies, which are described in detail below. lowing matrix: 2.5.2. Image Simulation 1−g −g A=(1−κ) 1 2 , (4) The translation of the measured ellipticity to the ap- (cid:18) −g2 1+g1 (cid:19) plied shear is not straightforward. First, a response to a shear depends on galaxy populations. This is because where κ is the projected mass density in units of the the changeinthe secondmoments undera givenshearγ critical lensing density and g is the reduced shear g = dependsnotonlyonthesecondmomentsthemselves,but γ/(1−κ). In the weak-lensing regime, κ is small and also on the higher moments (Mandelbaum et al. 2012). thus the γ ≃ g assumption is often made. The (1−κ) This makes the effects of morphological features such as factor affects the overall magnification, which is observ- radialprofiles,bulge-to-diskratios,spiralarms,etc. non- able through the measurement of bias in object num- negligible. As we model a galaxy light distribution with ber density or size distributions. The transformation an elliptical Gaussian in the current study, we should matrix shears a circle into an ellipse with an elliptic- understand how much the lack of details in the model ity g = (g2 + g2)1/2 = (1 − r)/(1 + r), where r is 1 2 biases the lensing signal. Second, ellipticity measure- the ratio of the semi-minor axis to the semi-major axis ment is a noisy process. As most lensing signals come (i.e., b/a). The position angle of the ellipse is given by from faint galaxies, this measurement noise significantly 1/2 tan−1(g /g ). 2 1 dilutesthesignal. Third,anontrivialfractionofgalaxies Using complex notation g = g +ig , we can also ex- 1 2 are affected by catastrophic shape measurement errors. press the ellipticity transformation when an object has The sources of these catastrophic shape errors include an initial ellipticity e=e +ie as 1 2 substructures of galaxies (e.g., HII regions), crowding, g+e “bleed” trails, clipped objects, galactic cirrus, spurious e′ = , (5) 1+g∗e detection around bright objects, etc. As the ellipticity measurement from these sources does not contain any wheretheasteriskrepresentscomplexconjugationande′ lensingsignal,the directionofthe biaswillalwaysbeto- is the measured ellipticity. If we assume that the distri- ward underestimation. In the current paper, instead of bution of e is isotropic, we can derive g from averaging quantifyingtheeffectofeachfactorseparately,wechoose over a population of galaxies using to derive a global value for shear responsivity R. Al- thoughitisworthinvestigatingtheeffectofeachfactorin 1 µ e′ g= i i, (6) isolation, marginalizing over other parameters increases RP µi the required number of simulated image sets consider- P ably, which is beyond the scope of the current study. where µ is a weight for each galaxy i. In the current i We utilize a modified version of the Large Synoptic paper, we use the following inverse variance as weight: Survey Telescope (LSST) image simulator presented in 1 Jee & Tyson (2011). The simulator samples galaxy im- µ = , (7) i σ2 +(δe )2 ages from the Hubble Space Telescope (HST) / Ultra SN i Deep Field (UDF; Beckwith et al. 2003) images and whereσ isashapenoiseofthepopulationpercompo- convolves them with the PSFs computed from the at- SN nent (∼0.25)andδei is the ellipticity measurementerror mospheric turbulence model and the telescope optics. per component. In equation 6, R is called the shear re- The purpose of this modified image simulator is to cal- sponsivity,whichis a calibrationfactornecessaryto rec- ibrate the conversion of ellipticity to shear. Given the oncile the difference between the average ellipticity and same galaxy profile, the size and intrinsic ellipticity of the shear. It is easy to show that R ≈ 1 if no mea- the PSF are the most important factors affecting this surementnoiseis presentandgalaxymorphologycanbe calibration parameter. The main difference in the PSF described by a simple elliptical isophote. However, be- between LSST and the two 4-m telescopes comes from causeneitheristrueintherealworld,onemustestimate 1)differentf-ratios(f/1.24andf/2.7 forLSST andMay- R withcare,andthis is one ofthe mostcriticalissues in all, respectively), 2) exposure time (15 s vs. 900 s), and future large lensing surveys since the result will not be 3) atmospheric seeing (0′.′65 versus 0′.′85). We address limited by statistical uncertainties. 2) and 3) by changing the atmospheric parameters (e.g., Ideally, it is desirable to estimate R analytically from Fried parameter and outer scale) in such a way that the first principles and use image simulations only to ver- resulting seeing distribution is close to the observation. ify the accuracy. Bernstein & Jarvis (2002) provided an Wecannotaddress1)directlywithoutreplacingthe cur- important contribution and their prescription has been rentLSSTopticaldesignmodelwiththemostup-to-date used in quite a few studies (e.g., Jarvis et al. 2006, Hi- Mayall/Blancotelescope models. However, it is possible rata et al. 2004). Nevertheless,more rigorousefforts are to approximate the effect by degrading the focus (and stillneededtoquantifytheapplicabilityandlimitsofthe optical alignment) so that when the diffraction limited Bernstein & Jarvis (2002)method. For the current DLS PSF is convolved with the atmospheric PSF, it matches cosmic shear analysis, we find that the shear responsiv- theDLSpattern. Withoutthisadjustment,thedelivered ity derived with the Bernstein & Jarvis (2002) method DLS PSF is severely circularized by atmosphere (longer agrees reasonably well with the value obtained from exposureandlargeatmosphericPSF). After this modifi- 10 Jee et al. Figure 9. Shear recovery test. The calibration factor (inverse slopeofthesolidlines)dependsontheS/Nofthesourcegalaxies. ThehighS/Ngalaxies(medianmagnitude∼23)requireacalibra- tionfactor of∼1.05whereas the calibrationfactor ofthe low S/N Figure 10. R-band magnitude versus shear calibration factor. galaxies(medianmagnitude∼26)isashighas∼1.28. Thedatapoints(diamond)aremeasuredfromimagesimulations. The black solid line represents our parameterization of the shear cation,weobtainadistributionofPSFellipticityranging calibration factor. The mean shear calibration factor derived by from 2% to 7%, matching the DLS data. Another im- multiplyingthiscurveandtheweightedmagnitudedistributionof sourcegalaxies(red)is∼1.08. portant question might be whether or not the resulting spatialvariationwithina single DLS CCDis realistic. If ofmagnitude. Figure9showstheresultsfortwoofthese oursimulatedPSFlacksasmallscalevariationcompared simulated populations where the mean apparent magni- tothatofthedata,thePSFmodelinthesimulationmay tudes are approximately 23 (open) and 26 (filled). We beeasiertodescribethaninrealsituations. Wefindthat omit the results from intermediate magnitude objects to theresidualPSFcorrelationforbothsimulationanddata avoidclutter. Theslopeofthelineistheshearresponsiv- shows a similar residual amplitude at all relevant scales, ity in equation 6. For the bright population, we obtain which suggests that the spatial variation of the PSF on R ∼ 0.95. This is similar to the value R ∼ 0.93 that both simulated images and DLS data possess a similar we obtain using eqn. 5.33 of Bernstein & Jarvis (2002). level of complexity. However, for the faint population the shear responsivity Mostlensingsignalscomefromz ∼1galaxies,theme- R∼0.78determined fromourimage simulationis lower dianofthen(z)counts,whicharedominatedbythefaint than the analytic estimate R∼0.89. Figure 10 summa- bluegalaxy(FBG)population. Hence,itisimportantto rizestheresultswhenwecombinetheresultsofthisshear test shear measurement from these UDF galaxies rather recovery test for four magnitude bins. We parameterize than from synthetic galaxies with analytic profiles. We thedependenceofthemultiplicativefactorm =1/Ron randomize both the orientation and the position of the γ the r−band magnitude (m ) with the following form: HST galaxiessothatthe netshearvanishes. We referto R theseimagesaszeroshearsky(ZSS).Inastrictsense,the m =6×10−4(m −20)3.26+1.036, (8) ZSSimagesarealreadyconvolvedbythe PSFoftheAd- γ R vanced Camera for Surveys, and thus are not the “true” wherem isSExtractor’sMAG AUTO.Inderivinganaver- R skyimagesintheabsenceoftheinstrumentseeing. How- age multiplicative factor hm i, we need to considerboth γ ever,becausethesizeoftheACSPSFisafactorofeight the magnitude and weight distributions of the source smaller, the effect in the creation of the final DLS im- population; the source selection criteria are discussed ages is limited to a scale far smaller than the DLS pixel in detail in §2.7. The magnitude distribution of our (∼0.257′′). Weapplyagravitationalshearusingbi-cubic source galaxies peaks at m ∼ 24 and then precipi- R interpolation to the ZSS images. As we have not down- touslydecreases(virtuallynogalaxiesbeyondm ∼26). R sampledthe UDF imagesyet,anyinterpolationartifacts In addition, smaller weights are given to ellipticities of andtheirpropagationareexpectedtobeinsignificanton faint galaxies (eqn. 6 and 7). The red histogram dis- the final image. Then, we convolve these sheared sky plays this weighted magnitude distribution. The re- (SS) images with spatially varying DLS PSFs. Readers sulting mean multiplicative factor is estimated to be are referred to Jee & Tyson (2011) for the details of the hm i=1.08±0.01. γ algorithminvolvedinthisstep. Finally,wedown-sample As this multiplicative factor is determined from the the convolvedsheared (CS) images and add noise to the galaxies in the UDF, it is possible that the above shear result in order to match the pixel scale and depth of our calibration may need to be refined further for the DLS DLS images. data. Indeed, our experience suggests that galaxy mor- Our goal is to determine the relation between input phology and size distributions are non-negligible factors shearsandweightedsumofthe ellipticities asa function in shear calibration. Currently, the UDF images are the
